



Next: Vector fields and the
Up: Differential Geometry. Honours 1996
Previous: Stokes theorem.
Contents
Partitions of unity.
If  is a manifold a partition of unity is a collection of smooth
non-negative functions
is a manifold a partition of unity is a collection of smooth
non-negative functions
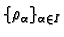 such that every
such that every 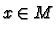 has neighbourhood
on which only a finite number of the
has neighbourhood
on which only a finite number of the 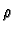 are
non-vanishing and such that
are
non-vanishing and such that
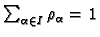 .
.
Recall that if
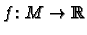 is smooth function
then we define
supp
is smooth function
then we define
supp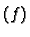 to be the closure of the set on which
to be the closure of the set on which 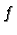 is
non-zero. There are two basic existence results on a paracompact,
Hausdorff manifold.
is
non-zero. There are two basic existence results on a paracompact,
Hausdorff manifold.
- 1.
- If
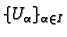 is an open cover
of
is an open cover
of  there is a partition of unity
there is a partition of unity
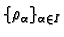 with
supp
with
supp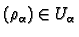 . Such a partition
of unity is called subordinate to the cover.
. Such a partition
of unity is called subordinate to the cover.
- 2.
- If
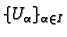 is an open cover
of
is an open cover
of  there is a partition of unity
there is a partition of unity
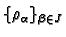 ,
with a possibly different indexing set
,
with a possibly different indexing set 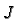 with each
supp
with each
supp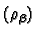 in some
in some
 .
.
Michael Murray
1998-09-16
![]() is smooth function
then we define
supp
is smooth function
then we define
supp![]() to be the closure of the set on which
to be the closure of the set on which ![]() is
non-zero. There are two basic existence results on a paracompact,
Hausdorff manifold.
is
non-zero. There are two basic existence results on a paracompact,
Hausdorff manifold.