



Next: Co-ordinate tangent vectors and
Up: The tangent space.
Previous: The tangent space.
Contents
Recall that a function
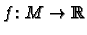 is
smooth if we can cover
is
smooth if we can cover  with co-ordinates
with co-ordinates 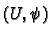 such that
such that
 is smooth .
If
is smooth .
If 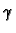 is a smooth path through
is a smooth path through 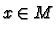 then if follows from the chain rule that
is smooth. Hence we can differentiate the function
then if follows from the chain rule that
is smooth. Hence we can differentiate the function
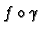 at
at 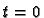 . By the chain rule we have that
It follows that
. By the chain rule we have that
It follows that
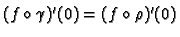 if
if 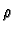 and
and 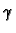 are in the same tangency class. Hence if
are in the same tangency class. Hence if
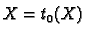 is a tangent vector in
is a tangent vector in 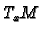 we can define
We call this the rate of change of
we can define
We call this the rate of change of 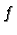 in the
direction
in the
direction 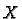 . Notice that we can calculate
. Notice that we can calculate
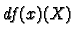 without explicit reference to the
path
without explicit reference to the
path 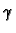 by the formula
As we vary the tangent vector
by the formula
As we vary the tangent vector 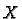 we define a map
called the differential of
we define a map
called the differential of 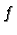 at
at 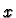 . This map satisfies the
formula
and hence, being a composition of linear maps,
is linear.
. This map satisfies the
formula
and hence, being a composition of linear maps,
is linear.
We call the set of linear maps from 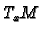 to
to
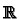 the cotangent space to
the cotangent space to  at
at 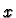 and denote it by
and denote it by  . So we have
. So we have
Elements of  are also called one-forms.
are also called one-forms.




Next: Co-ordinate tangent vectors and
Up: The tangent space.
Previous: The tangent space.
Contents
Michael Murray
1998-09-16
![]() to
to
![]() the cotangent space to
the cotangent space to ![]() at
at ![]() and denote it by
and denote it by ![]() . So we have
. So we have